Foucault’s pendulum

It was the morning hours of 3 January 1851 when Jean Bernard Léon Foucault became the first person — without looking up at the sky — to observe that the earth was turning. The 32-year-old French experimental physicist observed the oscillations of a two-metre-long thread pendulum in the cellar of his Paris house. He had experimented for many months to suspend this pendulum so that it could move almost frictionlessly. And so he noticed that the plane of oscillation of the pendulum (i.e. the plane in which the pendulum swings) appeared to rotate in relation to the floor of the cellar, by 11 degrees of arc per hour. It was the birth of the first terrestrial experiment that proved the rotation of the Earth without “a glance at the sky“. Since an external force acting on the pendulum could be ruled out, it was therefore not the pendulum but the floor of the cellar (i.e. the earth) that changed its direction.

Foucault later demonstrated the experiment at the Paris Observatory with a 12-metre-long pendulum and then (every Thursday from 31 March 1851) at the Paris Panthéon, the French Hall of Fame with the tombs of famous scientists, artists and statesmen, with a 67-metre-long pendulum and a 29-kg pendulum bob. The famous Italian writer Umberto Eco was inspired by this pendulum to write his world-famous novel “The Foucault Pendulum“.

So it is clear: the oscillation plane of Foucault’s pendulum only appears to rotate. In fact, it maintains its direction while you circle the pendulum on the globe. This phenomenon can be understood, for example, by the following mental experiment, if one imagines the pendulum suspended at the North Pole. Here the pendulum always swings in the same direction, while an observer on the rotating earth moves once in a circle on a day below it. The direction of oscillation consequently changes by 15 degrees per hour. In the direction of the equator, the hourly deviation decreases (here in Dresden — approximately at the 51st parallel 11.7 degrees per hour). At the equator, the axis of oscillation of the Foucault pendulum does not turn at all!

Below are the technical data and details of the Foucault pendulum in ADVENTURE LAND MATHEMATICS:

Latitude: $51^\circ 2' 31.5240''$ ( $51.04209^\circ$ )
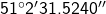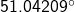There are 71 stones set up to illustrate the rotation.
Pendulum bob: ball bearing ball 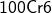$100\mathrm{Cr}6$ , diameter 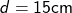$d=15\mathrm{cm}$ , mass approx. $13.8\mathrm{kg}$ .
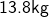Pendant rope: Dyneemad $4\mathrm{mm}$ .
Rope length $l\approx 18\mathrm m$ .
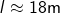Period of oscillation of the pendulum: 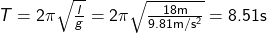$T=2\pi\sqrt{\frac{l}{g}}=2\pi\sqrt{\frac{18\mathrm m}{9.81\mathrm m/\mathrm s^2}}=8.51\mathrm s$ , with acceleration due to gravity $g=9.81\mathrm m/\mathrm s^2$ .
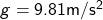Bearing: needle bearing, cardanic.

The Foucault pendulum was set up in ADVENTURE LAND MATHEMATICS with the kind support of the Dresden Max Planck Institute for Chemical Physics of Solids in an old lift shaft from the 3rd floor of the Technical Collections up to the 9th floor in the $48\mathrm{m}$ high Ernemannturm.

And now … the mathematics:

Only at the geographic poles — through which the Earth’s axis of rotation passes — does the pendulum’s plane of oscillation turn exactly $360^\circ$ in 24 hours. To be precise, it is 23 hours 56 minutes and 4 seconds ( $23.93\mathrm h$ ). That is the length of a starry day on Earth. The angular velocity of the rotation of the pendulum plane now decreases to zero up to the equator. The mathematical relationship is described with the sine of the latitude:

$\omega=\omega_0\sin(\varphi)$

with the angular velocity $\omega$ (in $^\circ/\mathrm h$ ), the angular velocity at the pole $\omega_0$ of $360^\circ/23,93\mathrm h\approx 15^\circ/\mathrm h$ , and the latitude $\varphi$ .

What is the situation at one pole? The latitude of the poles is $90^\circ$ . Therefore,

$\omega=\omega_0\sin(90^\circ)=\omega_0\approx 15^\circ/\mathrm h.$

The equator has the latitude zero. This also gives us

$\omega=\omega_0\sin(0^\circ)=0,$

so there is no rotation of the plane of oscillation at the equator.

The location of the Foucault pendulum in the ADVENTURE LAND MATHEMATICS of the Dresden Technical Collections in Junghansstraße is at the position $51.04209^\circ$ north latitude. How fast does our pendulum turn here in one hour? We get:

$\omega=\omega_0\sin(51,04209^\circ)\approx 11,7^\circ/\mathrm h.$

The 71 stones in the full circle of our experiment with the Foucault pendulum are placed at a distance of about $5.1^\circ$ from each other (see the following figure 1) and are knocked over by the swinging pendulum.

But how long do you have to wait until the next stone is knocked over by the pendulum?

In one hour, the pendulum turns by $11.7^\circ$ . So in 26 minutes the next stone should fall. — But that is not true, because the stones are on gap. If the pendulum knocks over a stone on one side, the next one falls on the opposite side. So the pendulum plane only has to rotate by about $2.55^\circ$ to hit the next stone. This takes about 13 minutes.