Möbius Street

The Möbius strip (also: Möbius loop or Möbius band) is a (two-dimensional) surface that has only one edge and only one side:

Figure 1: The Möbius strip

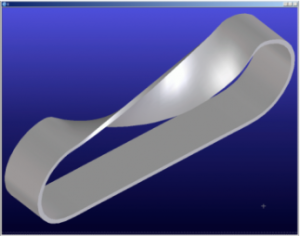
The Möbius strip was described independently in 1858 by the Göttingen professor of mathematics and physics, Johann Benedict Listing (1808–1892), and the astronomer August Ferdinand Möbius (1790–1868). Mathematically, it is a non-orientable manifold.

The construction of a Möbius strip can be understood in a simple way if one uses the comparable construction of a “normal” ring for comparison: Two long strips (of the same width) with parallel edges are cut out of a sheet of paper. In the first — the comparison strip — the two ends are smoothly joined together (e.g. glued) to form a “normal” ring. The second strip, the actual Möbius strip, is twisted half a turn (180°) before being joined. In this way, the Möbius strip becomes a fascinating little toy that can be easily made.

Now one can observe special phenomena: The ring-shaped twisted band, although it was created in a simple way from one strip of paper with an originally square edge and a clearly definable lower surface and surface, now has only one edge and one side.

Another way of looking at it leads to the conclusion that there is no “inside” and no “outside” on the Möbius strip, just as there is no “above” and “below“.

The following feature is also noteworthy:

A Möbius strip can be cut along its centre line without breaking into two separate rings half as wide as is automatically the case with the “normal” ring. The inner twist is then no longer only 180°, but 360°. If one repeats this cutting again, the Möbius strip disintegrates into two separate, intertwined individual strips.

In the visual arts, there are now famous depictions of the Möbius strip, e.g. that of the Dutch graphic artist Maurits Cornelis Escher (1963), the “Colossus of Frankfurt” by Max Bill (1908–1994) and the painting by the Dresden professor of geometry, Gert Bär (“Catastrophe in the Möbius strip“, 1968).

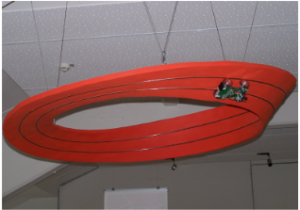
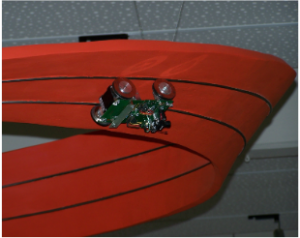
For illustration purposes, the Möbius strip in the Maths Adventure Land is driven on by a small vehicle, the Möbiusmobile (see Figure 1) (a technical masterpiece by the company …tronikDesign). It is also equipped with a mini camera whose images are continuously transmitted on a monitor. Incidentally, conveyor belts and drive belts are also manufactured as Möbius belts so that the supposed top or bottom side wears evenly.

Figure 2: The Möbius Mobile on the Möbius strip
Figure 3: The Möbius Street
Figure 4

And now … the mathematics:

The Möbius strip is a two-dimensional subset (area) in the three-dimensional space \mathbb R^3, which can be described by the following parameter representation:

    \[\begin {pmatrix} x\\ y\\ z\end {pmatrix} =\begin {pmatrix} \cos(\alpha)\left(1+\frac{r}{2}\cos(\alpha/2)\right)\\ \sin(\alpha)\left(1+\frac{r}{2}\cos(\alpha/2)\right)\\ \frac{r}{2}\sin(\alpha/2)\end{pmatrix}.\]

Here 0\leq\alpha\lt 2\pi (“orbital parameter“) and -1\lt r\lt 1 (“radial parameter“) apply.

Literature

[1] Greenland, C.: Begegnungen auf dem Möbiusband, Roman, München, 1996.

[2] Herges, R.: Möbius, Escher, Bach — Das unendliche Band in Kunst und Wissenschaft, in: Naturwissenschaftliche Rundschau (6/58), Darmstadt, 2005.

[3] Paenza, A.: Mathematik durch die Hintertür — Band 2: Vom Möbiusband zum Pascalschen Dreieick — neue spannende Ausflüge in die Welt der Zahlen, München, 2009.